Goodbye, Massachusetts

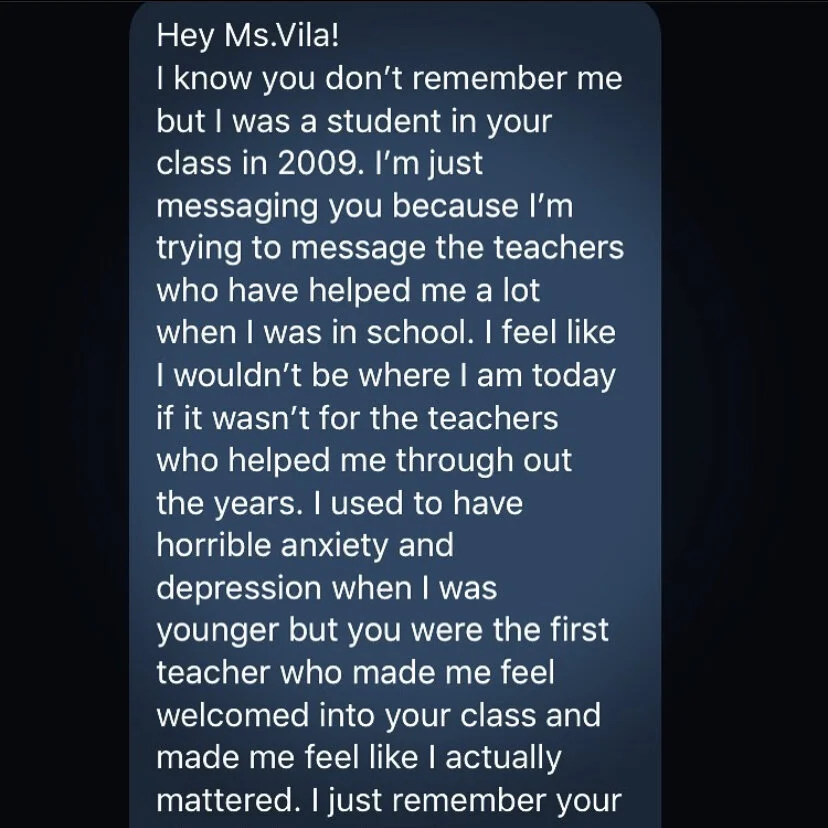
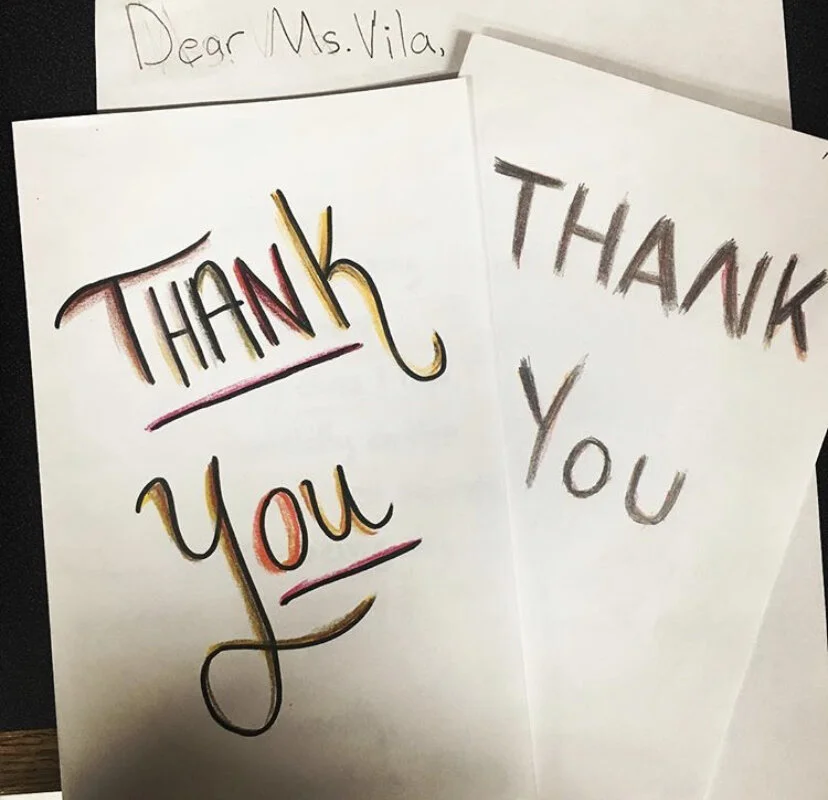My family and I have moved from Massachusetts to New York. Massachusetts, and specifically Medford MA where I taught 14 of the last 16 years, will always have a piece of my heart. But, it was time to move on.

I recently completed the NYC Online Teacher Application in which a short personal statement was required. I thought I’d share it here. Enjoy.

It is 2003 and I am looking at the stars on a clear night. "I want to change my major from mathematics to astrophysics," I think to myself. The universe is so beautiful, and as a young undergraduate I am simply fascinated. Although today I am still in awe of the night sky and all that lies behind it, I would not change my major during my time at Villanova University. Instead, I vowed to seek meaningful work that would directly impact those "down here" on Earth. I chose to help improve the society around me instead of keeping my head above the clouds. That was the moment I decided to become a math teacher.

Since 2007 when I graduated with a Bachelor's of Mathematics, I have taught thirteen years of middle school math and three years of high school math in my home state of Massachusetts. I have also gained two more degrees in education -- a master's and a doctorate -- to further improve my craft. I have helped students succeed in their coursework, perform well on important tests, and strengthen their love of learning.

I recently moved to New York. I currently seek employment as a middle school math teacher because that is the license that is transferable to New York. However, in Massachusetts I am also eligible to teach high school, which I am happy to do in New York if the opportunity arises. Regardless of grade level, I am searching for a new professional home: a community of learners that is diverse and energetic in both staff and student body. I work best with students who are unsure of themselves, but who are curious of the world around them (this, I have learned, can manifest itself in many ways!)

I am dedicated to both the art and science of teaching, and continue to read and network in order to grow professionally each year. I am communicative with guardians, eager to collaborate with colleagues, and extremely organized and reflective with my work. Most importantly, I believe in being a "warm demander" -- an educator who insists on asking students for their best effort and can do so without turning them away. This is but one of my strengths.

I look forward to sharing more with you.

That Back-To-School Feeling

Hello friends,

I’ve been going back and forth on what to write about for this post. There’s so much going on in the world, in education, in life… I’m feeling a bit all over the place lately. I can’t seem to organize my thoughts as well as I like to think I usually do.

That being said, one thing that is clear is the excitement I feel during this last week of summer break. Each year, I’m sad summer is unofficially over (no more sleeping in!) but I’m also very much looking forward to meeting my new students and getting back into a routine. This year is no different.

As educators, how lucky are we that we get to start anew each school year? We can reflect on the previous year and make modifications as needed, forever reflecting, improving, failing, succeeding, and learning. Many professions don’t get such a clear period of reset, one between the old and the new. For that I am grateful.

Ahhh, that back-to-school feeling is special indeed. Don’t you agree?

Replicating the Zoom Chat Feature

My goodness, it has been too long. I hope you are doing well, reader. I’m in the home stretch of this crazy school year and have been in a reflective mood lately. Top of mind at this moment is Zoom’s chat feature. Let me explain.

It took a few weeks, but I think I fell into a nice rhythm using Zoom this year. I love both the chat and breakout room features. The chat feature allows me to check in with students without anyone else knowing. Conversely, students can ask a question (or many) without fear of “looking dumb” or being that person who asks something which was already answered.

I tried leaving it up to students whether they wanted to send a comment or question directly to me or to the whole class, but this did not work. Students would sometimes ask a private question publicly or give an answer to the whole class followed by a, “Oops, I meant to send that only to you, Ms. Vila.” I decided to change my Zoom settings so students can only direct message me. It’s not ideal, but I think it’s working pretty well. I often say, “Question in the chat…” and then proceed to state the question and, if needed, answer the question or allow others to help answer it. Students have mentioned that they like this method.

As of this writing, the plan is for all students to be back in the classroom next year. If that’s the case, how do I replicate the Zoom chat feature in school? If each student has a device, I suppose we can all log into a Zoom call… but would that work alright if we are all in the same room and I’m moving about? What if my district is not able to maintain the one-to-one Chromebook pairing that went on this year? These are questions I will try to answer this summer.

Biden's Pick for Secretary of Education

Yesterday, Joe Biden was inaugurated as the 46th president of the United States. I’m eager to see what happens — and does not happen — during his term. Naturally, I was curious about Biden’s pick for Secretary of Education. Although not yet confirmed, Biden has selected Miguel Cardona to America’s top education position. Cardona is currently Connecticut’s Commissioner of Education and is a former teacher. Here are two articles on Miguel Cardona:

• https://www.the74million.org/article/cardonas-role-in-connecticuts-complex-school-desegregation-efforts-becomes-focus-will-he-give-integration-a-national-platform-as-ed-secretary/

• https://www.usnews.com/news/education-news/articles/2020-12-30/miguel-cardona-bidens-pick-for-education-secretary-stares-down-a-long-to-do-list

My First Podcast Episode!

Back in October I had the opportunity to appear on The Dr. Will Show podcast. The episode focused on my tutoring business, but we also discussed my research and teaching experience. It was a lot of fun and although it was my first time appearing on a podcast, I hope it’s not my last. Check it out here.

L4E

Since the summertime, I’ve been a part of a group of fellows selected by Latinos for Education. Latinos for Education, or L4E, is an organization that seeks to “develop, place and connect essential Latino leaders in the education sector” and “break down barriers to educational opportunity for the next generation of Latino students.” As part of the year-long fellowship, a group of about 20 Latinx individuals currently living in the Boston area meet once a month to engage in (Zoom) discussions and presentations relevant to the organization’s mission.

This month’s meeting focused on advocacy and I was greatly impressed with the facilitators. Manny Cruz, a new addition to Latinos for Education’s team, led or participated in multiple sessions throughout the day. Manny is a school committee member in Salem, Massachusetts and has experience working for two former State Representatives. He is an engaging and inspiring speaker, the kind of person who makes you think, “Should I run for School Committee?” and “How can I be more involved?” and “How can I generally be more like Manny?”

Overall, I’m thrilled to be a part of this group. It’s nice to reconnect with like-minded adults in a professional setting again. Remote teaching and canceled conferences has deprived me of this for many months now.

Meet the 2020 Massachusetts fellows here.

Source of mission, pictures, and stats above: https://www.latinosforeducation.org/.

Grasping Large Numbers

It’s difficult for humans to grasp large numbers. We just had no need to do so in our evolutionary past. Today, however, understanding large numbers is useful to forming an accurate picture of the world around us. From the national debt to populations of nations to distances between cities and planets, large numbers are now a more relevant part of our lives.

To date, the COVID-19 pandemic has killed about 1,120,000 people worldwide. Here in the United States, about 220,000 have passed, equaling about 20% of all who have passed due to the virus (the U.S. has about 4% of the world’s population.) Since 220,000 is a large number, I’d like to show how much 220,000 really is. Here are 220,000 dots.

Learn more: https://io9.gizmodo.com/how-to-comprehend-incomprehensibly-large-numbers-1531604757

My Favorite Math Theorem

Although I’ve always enjoyed math class, growing up I wasn’t someone who necessarily wanted to be a mathematician or a math teacher. I also wasn’t someone who pictured herself decades later blogging about her favorite math theorem. But here we are.

My favorite math theorem is the Borsuk-Ulam Theorem. Here’s an explanation from Mudd Math Fun Facts:

“The Borsuk-Ulam theorem is another amazing theorem from topology. An informal version of the theorem says that at any given moment on the earth’s surface, there exist 2 antipodal points (on exactly opposite sides of the earth) with the same temperature and barometric pressure!

More formally, it says that any continuous function from an n-sphere to R^n must send a pair of antipodal points to the same point. (So, in the above statement, we are assuming that temperature and barometric pressure are continuous functions.)”

It goes on to explain the simplest iteration of this theorem:

“Show your students the 1-dimensional version: on the equator, there must exist opposite points with the same temperature. Draw a few pictures of possible temperature distributions to convince them that it is true.”

Think about that. There HAS to be two opposite points on the Earth’s equator with the same exact temperature. Woah. Here’s how we know it’s true:

“The one dimensional proof gives some idea why the theorem is true: if you compare opposite points A and B on the equator, suppose A starts out warmer than B. As you move A and B together around the equator, you will move A into B’s original position, and simultaneously B into A’s original position. But by that point A must be cooler than B. So somewhere in between (appealing to continuity) they must have been the same temperature!”

Isn’t that cool?

Citation: Su, Francis E., et al. “Borsuk-Ulam Theorem.” Math Fun Facts. <https://www.math.hmc.edu/funfacts>.

Preparing for a Remote Start

A few days ago, my district of Medford, MA voted to start the school year remotely. Although I’ve been curating remote teaching tools all summer, I only recently dove deep into my email folders and Twitter bookmarks to figure out whether anything I’d collected might be useful in my day-to-day teaching this fall. Here’s what I found that I believe is worth a share:

Synchronous teaching:

• Since Medford is a Google district, I plan on using Google Slides to help organize my synchronous lessons. Even if it’s one slide with the agenda and maybe a link or two, I think creating and sharing this with students will be helpful and help me stay organized. For more involved lessons there’s a lot you can do with Google Slides that I recently discovered. If you’re planning on using Slides too, here’s a video with add-on recommendations. Here’s a massive collection of slide templates as well.

• I’ve come across Whiteboard.fi and can see myself using it in class. It allows a teacher to present a problem on a digital whiteboard and then gives students their own whiteboard to show work and/or answers. The teacher can then see each student whiteboard but students cannot see each other’s. Here’s an intro video to learn more.

• Another whiteboard resource is Ziteboard. This one seems to be better for direct instruction and student collaboration versus Whiteboard.fi which seems better for practice. It came highly recommended by another educator and I’m looking forward to testing it out.

• Another popular collaboration and whiteboard resource is Jamboard. Many teachers love this one. It seems to help teachers create engaging lessons, easy to save for future use and easy to share with students. Here’s a how-to video.

Asynchronous teaching:

• I plan on having my students use a few different sites and tools for asynchronous learning. The main site I’ll use will be the Houghton Mifflin Harcourt (HMH) site that is the online component of the math program purchased by my district.

• Typically, though, one program’s online component is not enough. For further independent practice I plan on utilizing sites like Khan Academy, DeltaMath, and Desmos. (DeltaMath and Desmos would be useful for math teachers only.) Although I’m familiar with Khan Academy and have used it in class before, I will have to learn more about DeltaMath and Desmos. Many math teachers I know use both regularly.

• Loom is a neat application I discovered that allows you to record your screen with your face in the bottom corner for a more personal video experience. I’ve tested it out and love how easy it is to use. I plan on using it for a “welcome” video. I hope to also use it to make videos of myself modeling math problems or giving students directions for asynchronous assignments.

Other:

• I believe all of the above is free. Last night, however, I caved and bought myself a new desk and chair. Here are some other items on my wishlist: monitor for dual screening, microphone to ensure high sound quality for my students, document camera, iPad & Apple Pencil. As with the online tools, these tech items were recommended by other teachers.

• Lastly, two coworkers and I have created a document to organize the resources we’ve found. Here it is if you’re interested. I plan on using this document to do a bit more research into what may possibly help me teach from home this upcoming year.

Goodbye, Middle School

After 13 years teaching middle school students, I’m transitioning to teaching high school math starting this fall.

The change is bittersweet. I’m looking forward to trying something new, but I will miss middle schoolers and everything that comes with teaching them.

Here are some of my favorite pictures from over the years:

Coworkers who helped me along the way:

Things that made me smile, laugh, or both.

Sweet notes:

Field trips and other special days including a visit from writer Jonny Sun:

Goodbye, middle school!